State, whether the following statements are true or false. If false, give a reason.
(i) If A and B are two matrices of orders 3 ![]() 2 and 2
2 and 2 ![]() 3 respectively; then their sum A + B is possible.
3 respectively; then their sum A + B is possible.
(ii) The matrices ![]() and
and ![]() are conformable for subtraction.
are conformable for subtraction.
(iii) Transpose of a 2 ![]() 1 matrix is a 2
1 matrix is a 2 ![]() 1 matrix.
1 matrix.
(iv) Transpose of a square matrix is a square matrix.
(v) A column matrix has many columns and one row.
(i) False
The sum A + B is possible when the order of both the matrices A and B are same.
(ii) True
(iii) False
Transpose of a 2 ![]() 1 matrix is a 1
1 matrix is a 1 ![]() 2 matrix.
2 matrix.
(iv) True
(v) False
A column matrix has only one column and many rows.
Given: ![]() , find x, y and z.
, find x, y and z.
If two matrices are equal, then their corresponding elements are also equal. Therefore, we have:
x = 3,
y + 2 = 1 ![]() y = -1
y = -1
z - 1 = 2 ![]() z = 3
z = 3
Solve for a, b and c if
(i) ![]()
(ii) ![]()
If two matrices are equal, then their corresponding elements are also equal.
(i)
a + 5 = 2 ![]() a = -3
a = -3
-4 = b + 4 ![]() b = -8
b = -8
2 = c - 1 ![]() c = 3
c = 3
(ii) a= 3
a - b = -1
![]() b = a + 1 = 4
b = a + 1 = 4
b + c = 2
![]() c = 2 - b = 2 - 4 = -2
c = 2 - b = 2 - 4 = -2
If A =![]() and B =
and B = ![]() ; find: (i) A + B (ii) B - A
; find: (i) A + B (ii) B - A
(i) A + B =![]()
(ii) B - A ![]()
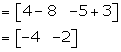
If A=![]() , B =
, B = ![]() and C =
and C = ![]() ; find:
; find:
(i) B + C (ii) A - C
(iii) A + B - C (iv) A - B +C
(i)B + C = ![]()
(ii)A - C = ![]()
(iii)A + B - C =![]()
=![]() =
= ![]()
(iv)A - B +C =![]()
=![]() =
= ![]()
Wherever possible, write each of the following as a single matrix.
(i) ![]()
(ii)
![]()
(iii)
![]()
(i) ![]()
(ii) ![]()
(iii) Addition is not possible, because both matrices are not of same order.
Find, x and y from the following equations :
![]()
![]()
(i)

Equating the corresponding elements, we get,
3 - x = 7 and y + 2 = 2
Thus, we get, x = - 4 and y = 0.
(ii)
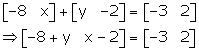
Equating the corresponding elements, we get,
-8 + y = -3 and x - 2 =2
Thus, we get, x = 4 and y = 5.
Given: M =![]() , find its transpose matrix Mt. If possible, find:
, find its transpose matrix Mt. If possible, find:
(i) M + Mt (ii) Mt - M
M =![]()
Mt = ![]()
(i) ![]()
(i) ![]()
Write the additive inverse of matrices A, B and C:
Where ![]()
We know additive inverse of a matrix is its negative.
Additive inverse of A = ![]()
Additive inverse of B = ![]()
Additive inverse of C = ![]()
Given ![]() ; find the matrix X in each of the following:
; find the matrix X in each of the following:
(i) X + B = C - A
(ii) A - X = B + C
(i) X + B = C - A
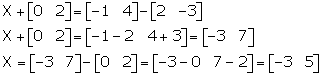
(ii) A - X = B + C
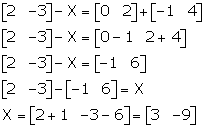
Given ![]() ; find the matrix X in each of the following:
; find the matrix X in each of the following:
(i) A + X = B
(ii) A - X = B
(iii) X - B = A
(i) A + X = B
X = B - A
![]()
(ii) A - X = B
X = A - B
![]()
(iii) X - B = A
X = A + B
![]()
No comments:
Post a Comment