Find, in each case, the remainder when:
By remainder theorem we know that when a polynomial f (x) is divided by x - a, then the remainder is f(a).
Show that:
(x - a) is a factor of a polynomial f(x) if the remainder, when f(x) is divided by (x - a), is 0, i.e., if f(a) = 0.
Use the Remainder Theorem to find which of the following is a factor of 2x3 + 3x2 - 5x - 6.
(i) x + 1
(ii) 2x - 1
(iii) x + 2
By remainder theorem we know that when a polynomial f (x) is divided by x - a, then the remainder is f(a).
Let f(x) = 2x3 + 3x2 - 5x - 6
(i) f (-1) = 2(-1)3 + 3(-1)2 - 5(-1) - 6 = -2 + 3 + 5 - 6 = 0
Thus, (x + 1) is a factor of the polynomial f(x).
(ii)

Thus, (2x - 1) is not a factor of the polynomial f(x).
(iii) f (-2) = 2(-2)3 + 3(-2)2 - 5(-2) - 6 = -16 + 12 + 10 - 6 = 0
Thus, (x + 2) is a factor of the polynomial f(x).
(i) If 2x + 1 is a factor of 2x2 + ax - 3, find the value of a.
(ii) Find the value of k, if 3x - 4 is a factor of expression 3x2 + 2x - k.
(i) 2x + 1 is a factor of f(x) = 2x2 + ax - 3.

(ii) 3x - 4 is a factor of g(x) = 3x2 + 2x - k.
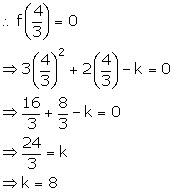
Find the values of constants a and b when x - 2 and x + 3 both are the factors of expression x3 + ax2 + bx - 12.
Let f(x) = x3 + ax2 + bx - 12
x - 2 = 0 ![]() x = 2
x = 2
x - 2 is a factor of f(x). So, remainder = 0
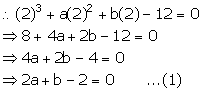
x + 3 = 0 ![]() x = -3
x = -3
x + 3 is a factor of f(x). So, remainder = 0
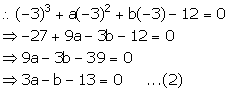
Adding (1) and (2), we get,
5a - 15 = 0
![]() a = 3
a = 3
Putting the value of a in (1), we get,
6 + b - 2 = 0
![]() b = -4
b = -4
find the value of k, if 2x + 1 is a factor of (3k + 2)x3 + (k - 1).
Let f(x) = (3k + 2)x3 + (k - 1)
2x + 1 = 0 ![]()
![]()
Since, 2x + 1 is a factor of f(x), remainder is 0.
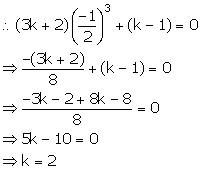
f(x) = 2x5 - 6x4 - 2ax3 + 6ax2 + 4ax + 8
x - 2 = 0 ![]() x = 2
x = 2
Since, x - 2 is a factor of f(x), remainder = 0.
2(2)5 - 6(2)4 - 2a(2)3 + 6a(2)2 + 4a(2) + 8 = 0
64 - 96 - 16a + 24a + 8a + 8 = 0
-24 + 16a = 0
16a = 24
a = 1.5
Let f(x) = x3 + (3m + 1) x2 + nx - 18
x - 1 = 0 ![]() x = 1
x = 1
x - 1 is a factor of f(x). So, remainder = 0
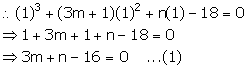
x + 2 = 0 ![]() x = -2
x = -2
x + 2 is a factor of f(x). So, remainder = 0
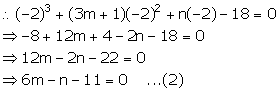
Adding (1) and (2), we get,
9m - 27 = 0
m = 3
Putting the value of m in (1), we get,
3(3) + n - 16 =0
9 + n - 16 = 0
n = 7
When x3 + 2x2 - kx + 4 is divided by x - 2, the remainder is k. Find the value of constant k.
Let f(x) = x3 + 2x2 - kx + 4
x - 2 = 0 ![]() x = 2
x = 2
On dividing f(x) by x - 2, it leaves a remainder k.
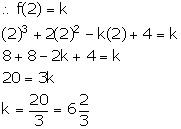
Find the value of a, if the division of ax3 + 9x2 + 4x - 10 by x + 3 leaves a remainder 5.
Let f(x) = ax3 + 9x2 + 4x - 10
x + 3 = 0 ![]() x = -3
x = -3
On dividing f(x) by x + 3, it leaves a remainder 5.
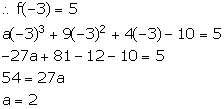
Let f(x) = x3 + ax2 + bx + 6
x - 2 = 0 ![]() x = 2
x = 2
Since, x - 2 is a factor, remainder = 0

x - 3 = 0 ![]() x = 3
x = 3
On dividing f(x) by x - 3, it leaves a remainder 3.
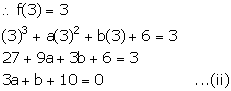
Subtracting (i) from (ii), we get,
a + 3 = 0
a = -3
Substituting the value of a in (i), we get,
-6 + b + 7 = 0
b = -1
The expression 2x3 + ax2 + bx - 2 leaves remainder 7 and 0 when divided by 2x - 3 and x + 2 respectively. Calculate the values of a and b.
Let f(x) = 2x3 + ax2 + bx - 2
2x - 3 = 0 ![]() x =
x = ![]()
On dividing f(x) by 2x - 3, it leaves a remainder 7.

x + 2 = 0 ![]() x = -2
x = -2
On dividing f(x) by x + 2, it leaves a remainder 0.
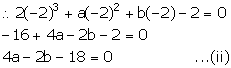
Adding (i) and (ii), we get,
7a - 21 = 0
a = 3
Substituting the value of a in (i), we get,
![]()
9 + 2b - 3 = 0
2b = -6
b = -3
What number should be added to 3x3 - 5x2 + 6x so that when resulting polynomial is divided by x - 3, the remainder is 8?
Let the number k be added and the resulting polynomial be f(x).
So, f(x) = 3x3 - 5x2 + 6x + k
It is given that when f(x) is divided by (x - 3), the remainder is 8.
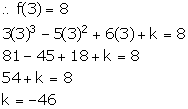
Thus, the required number is -46.
What number should be subtracted from x3 + 3x2 - 8x + 14 so that on dividing it with x - 2, the remainder is 10.
Let the number to be subtracted be k and the resulting polynomial be f(x).
So, f(x) = x3 + 3x2 - 8x + 14 - k
It is given that when f(x) is divided by (x - 2), the remainder is 10.
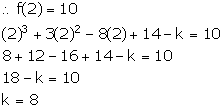
Thus, the required number is 8.
The polynomials 2x3 - 7x2 + ax - 6 and x3 - 8x2 + (2a + 1)x - 16 leaves the same remainder when divided by x - 2. Find the value of 'a'.
Let f(x) = 2x3 - 7x2 + ax - 6
x - 2 = 0 ![]() x = 2
x = 2
When f(x) is divided by (x - 2), remainder = f(2)
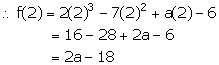
Let g(x) = x3 - 8x2 + (2a + 1)x - 16
When g(x) is divided by (x - 2), remainder = g(2)
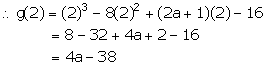
By the given condition, we have:
f(2) = g(2)
2a - 18 = 4a - 38
4a - 2a = 38 - 18
2a = 20
a = 10
Thus, the value of a is 10.
If (x - 2) is a factor of the expression 2x3 + ax2 + bx - 14 and when the expression is divided by (x - 3), it leaves a remainder 52, find the values of a and b
Find 'a' if the two polynomials ax3 + 3x2 - 9 and 2x3 + 4x + a, leave the same remainder when divided by x + 3.
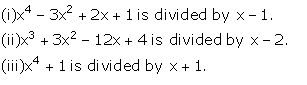
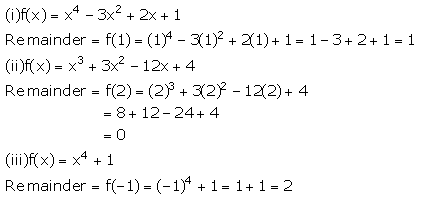


No comments:
Post a Comment