Chapter 6 - Solving (simple) Problems (Based on Quadratic Equations) Exercise Ex. 6(E)

Question 1

The distance by road between two towns A and B is 216 km, and by rail it is 208 km. A car travels at a speed of x km/hr and the train travels at a speed which is 16 km/hr faster than the car. Calculate:

(i) the time taken by the car to reach town B from A, in terms of x;

(ii) the time taken by the train to reach town B from A, in terms of x.

(iii) If the train takes 2 hours less than the car, to reach town B, obtain an equation in x and solve it.

(iv) Hence, find the speed of the train

Solution 1

Question 2

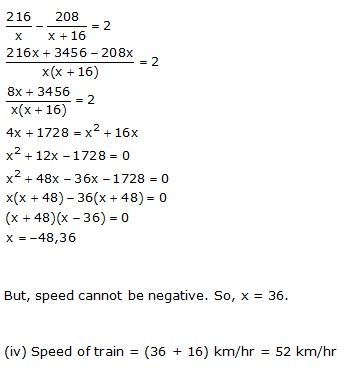
A trader buys x articles for a total cost of Rs 600.

(i) Write down the cost of one article in terms of x.

If the cost per article were Rs 5 more, the number of articles that can be bought for Rs 600 would be four less.

(ii) Write down the equation in x for the above situation and solve it for x.

Solution 2

Question 3

A hotel bill for a number of people for overnight stay is Rs 4800. If there were 4 people more, the bill each person had to pay, would have reduced by Rs 200. Find the number of people staying overnight.

Solution 3

Question 4

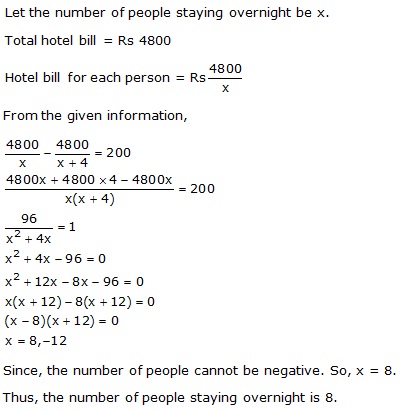
An Aero plane travelled a distance of 400 km at an average speed of x km/hr. On the return journey, the speed was increased by 40 km/hr. Write down an expression for the time taken for:

(i) the onward journey;

(ii) the return journey.

If the return journey took 30 minutes less than the onward journey, write down an equation in x and find its value.

Solution 4

Question 5

Rs 6500 was divided equally among a certain number of persons. Had there been 15 persons more, each would have got Rs 30 less. Find the original number of persons.

Solution 5

Question 6

A plane left 30 minutes later than the schedule time and in order to reach its destination 1500 km away in time, it has to increase its speed by 250 km/hr from its usual speed. Find its usual speed.

Solution 6

Question 7

Two trains leave a railway station at the same time. The first train travels due west and the second train due north. The first train travels 5 km/hr faster than the second train. If after 2 hours, they are 50 km apart, find the speed of each train.

Solution 7

Question 8

The sum S of first n even natural numbers is given by the relation S = n(n + 1). Find n, if the sum is 420.

Solution 8

S = n(n + 1)

Given, S = 420

n(n + 1) = 420

n² + n - 420 = 0

n² + 21n - 20n - 420 = 0

n(n + 21) - 20(n + 21) = 0

(n + 21) (n - 20) = 0

n = -21, 20

Since, n cannot be negative.

Hence, n = 20.

Question 9

The sum of the ages of a father and his son is 45 years. Five years ago, the product of their ages (in years) was 124. Determine their present ages.

Solution 9

Let the present ages of father and his son be x years and (45 - x) years respectively.

 Five years ago,

Father's age = (x - 5) years

Son's age = (45 - x - 5) years = (40 - x) years

 From the given information, we have:

(x - 5) (40 - x) = 124

40x - x² - 200 + 5x = 124

x² - 45x +324 = 0

x² - 36x - 9x +324 = 0

x(x - 36) - 9(x - 36) = 0

(x - 36) (x - 9) = 0

x = 36, 9

 If x = 9,

Father's age = 9 years, Son's age = (45 - x) = 36 years

This is not possible.

Hence, x = 36

Father's age = 36 years

Son's age = (45 - 36) years = 9 years

Question 10

In an auditorium, seats were arranged in rows and columns. The number of rows was equal to the number of seats in each row. When the number of rows was doubled and the number of seats in each row was reduced by 10, the total number of seats increased by 300. Find:

(i) the number of rows in the original arrangement.

(ii) the number of seats in the auditorium after re-arrangement.

Solution 10

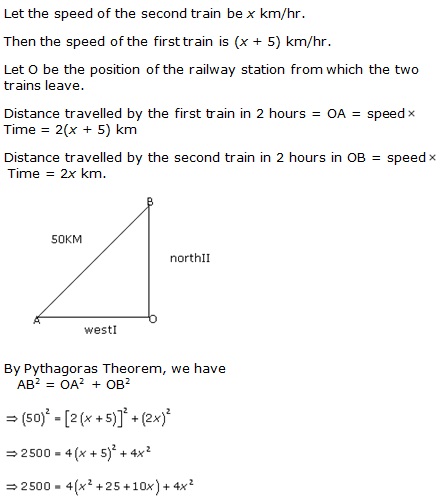
Let the number of rows in the original arrangement be x.

Then, the number of seats in each row in original arrangement = x

Total number of seats =

 From the given information,

2x(x - 10) = x² + 300

2x² - 20x = x² + 300

x² - 20x - 300 = 0

(x - 30) (x + 10) = 0

x = 30, -10

 Since, the number of rows or seats cannot be negative. So, x = 30.

 (i) The number of rows in the original arrangement = x = 30

(ii) The number of seats after re-arrangement = x² + 300 = 900 + 300 = 1200

Question 11

Mohan takes 16 days less than Manoj to do a piece of work. If both working together can do it in 15 days, in how many days will Mohan alone complete the work?

Solution 11

Question 12

Two years ago, a man's age was three times the square of his son's age. In three years time, his age will be four times his son's age. Find their present ages.

Solution 12

Let the age of son 2 years ago be x years.

Then, father's age 2 years ago = 3x² years

 

Present age of son = (x + 2) years

Present age of father = (3x² + 2) years

 

3 years hence:

Son's age = (x + 2 + 3) years = (x + 5) years

Father's age = (3x² + 2 + 3) years = (3x² + 5) years

 

From the given information,

3x² + 5 = 4(x + 5)

3x² - 4x - 15 = 0

3x² - 9x + 5x - 15 = 0

3x(x - 3) + 5(x - 3) = 0

(x - 3) (3x + 5) = 0

x = 3,

Since, age cannot be negative. So, x = 3.

 

Present age of son = (x + 2) years = 5 years

Present age of father = (3x² + 2) years = 29 years

Question 13

In a certain positive fraction, the denominator is greater than the numerator by 3. If 1 is subtracted from the numerator and the denominator both, the fraction reduces by 1/14. Find the fraction.

Solution 13

Question 14

In a two digit number, the ten's digit is bigger. The product of the digits is 27 and the difference between two digits is 6. Find the number.

Solution 14

Given, the difference between two digits is 6 and the ten's digit is bigger than the unit's digit.

So, let the unit's digit be x and ten's digit be (x + 6).

 

From the given condition, we have:

x(x + 6) = 27

x² + 6x - 27 = 0

x² + 9x - 3x - 27 = 0

x(x + 9) - 3(x + 9) = 0

(x + 9) (x - 3) = 0

x = -9, 3

 

Since, the digits of a number cannot be negative. So, x = 3.

 

Unit's digit = 3

Ten's digit = 9

 

Thus, the number is 93.

Question 15

Some school children went on an excursion by a bus to a picnic spot at a distance of 300 km. While returning, it was raining and the bus had to reduce its speed by 5 km/hr and it took two hours longer for returning. Find the time taken to return.

Solution 15

Question 16

Rs.480 is divided equally among 'x' children. If the number of children were 20 more, then each would have got Rs.12 less. Find 'x'.

Solution 16

Question 17

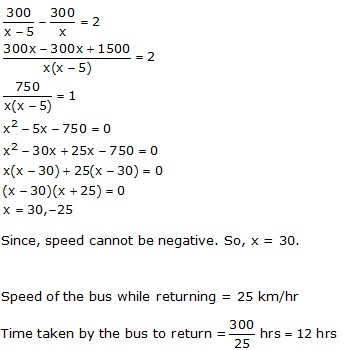
A bus covers a distance of 240 km at a uniform speed. Due to heavy rain its speed gets reduced by 10km/h and as such it takes two hrs longer to cover the total distance. Assuming the uniform speed to be 'x' km/h, form an equation and solve it to evaluate 'x'.

Solution 17

Time taken by bus to cover total distance with speed x km/h =  

Time taken by bus to cover total distance with speed (x - 10) km/h =  

According to the given condition, we have 

 

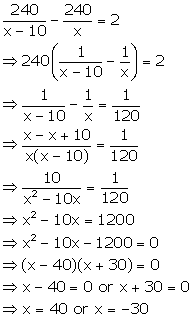
Since the speed cannot be negative, we have x = 40 km/h

Question 18

The sum of the ages of Vivek and his younger brother Amit is 47 years. The product of their ages in years is 550. Find their ages.

Solution 18

Given that he sum of the ages of Vivek and his younger brother Amit is 47 years.

Let the age of Vivek = x

⇒ the age of Amit = 47 - x 

The product of their ages in years is 550 …. given

⇒ x(47 - x) = 550

⇒ 47x - x² = 550

⇒ x² - 47x + 550 = 0  

⇒ x² - 25x - 22x  + 550 = 0

⇒ x(x - 25) - 22(x - 25) = 0

⇒ (x - 25) (x - 22) = 0

⇒ x = 25 or x = 22

Given that Vivek is an elder brother.

∴ x = 25 years = age of Vivek and

age of Amit = 47 - 25 = 22 years