Chapter 16 - Loci (Locus and its Constructions) Exercise Ex. 16(A)

Question 1

Given: PQ is a perpendicular bisector of side AB of the triangle ABC.

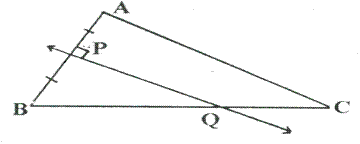
Prove: Q is equidistant from A and B.

Solution 1

Construction: Join AQ

Proof: In and ,

AP = BP (given)

 (Each = 90)

PQ = PQ (Common)

Hence Q is equidistant from A and B.

Question 2

Given: CP is the bisector of angle C of 

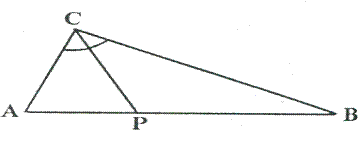
Prove: P is equidistant from AC and BC.

Solution 2

Construction: From P, draw and 

Proof: In and ,

Hence, P is equidistant from AC and AB

Question 3

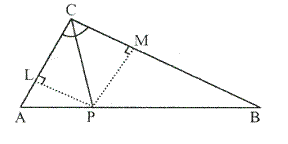
Given: AX bisects angle BAC and PQ is perpendicular bisector of AC which meets AX at point Y.

Prove:

i) X is equidistant from AB and AC.

ii) Y is equidistant from A and C

Solution 3

Construction: From X, draw and . Also join YC.

Proof:

i) In and ,

Hence, X is equidistant from AB and AC.

ii) In and ,

Hence, Y is equidistant from A and C.

Question 4

Construct a triangle ABC, in which AB = 4.2 cm, BC = 6.3 cm and AC = 5 cm. Draw perpendicular bisector of BC which meets AC at point D. Prove that D is equidistant from B and C.

Solution 4

Given: In triangle ABC, AB = 4.2 cm, BC = 6.3 cm and AC = 5 cm

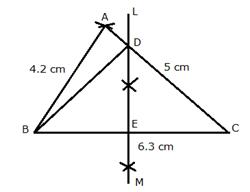
Steps of Construction:

i) Draw a line segment BC = 6.3 cm

ii) With centre B and radius 4.2 cm, draw an arc.

iii) With centre C and radius 5 cm, draw another arc which intersects the first arc at A.

iv) Join AB and AC.

 is the required triangle.

v) Again with centre B and C and radius greater than , draw arcs which intersects each other at L and M.

vi) Join LM intersecting AC at D and BC at E.

vii) Join DB.

Proof: In and 

Hence, D is equidistant from B and C.

Question 5

In each of the given figures: PA = PB and QA = QB

i)

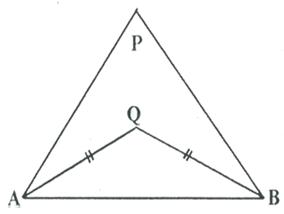
ii)

Prove in each case, that PQ (produced, if required) is perpendicular bisector of AB.

Hence, state the locus of the points equidistant from two given fixed points.

Solution 5

Construction: Join PQ which meets AB in D.

 

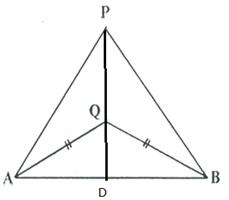
Proof: P is equidistant from A and B.

P lies on the perpendicular bisector of AB.

Similarly, Q is equidistant from A and B.

 Q lies on perpendicular bisector of AB.

 P and Q both lie on the perpendicular bisector of AB.

 PQ is perpendicular bisector of AB.

Hence, locus of the points which are equidistant from two fixed points, is a perpendicular bisector of the line joining the fixed points.

Question 6

Construct a right angled triangle PQR, in which , hypotenuse PR = 8 cm and QR = 4.5 cm. Draw bisector of angle PQR and let it meet PR at point T. Prove that T is equidistant from PQ and QR.

Solution 6

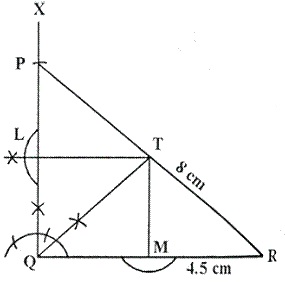
Steps of Construction:

i) Draw a line segment QR = 4.5 cm

ii) At Q, draw a ray QX making an angle of 

iii) With centre R and radius 8 cm, draw an arc which intersects QX at P.

iv) Join RP.

is the required triangle.

v) Draw the bisector of which meets PR in T.

vi) From T, draw perpendicular PL and PM respectively on PQ and QR.

Proof: In and 

Hence, T is equidistant from PQ and QR.

Question 7

Construct a triangle ABC in which angle , AB = 5cm and BC = 6.4 cm. Draw perpendicular bisector of side BC and also the bisector of angle ACB. If these bisectors intersect each other at point P; prove that P is equidistant from B and C; and also from AC and BC.

Solution 7

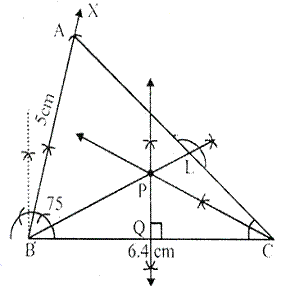
Steps of Construction:

i) Draw a line segment BC = 6.4 cm

ii) At B, draw a ray BX making an angle of  with BC and cut off BA = 5 cm.

iii) Join AC.

 is the required triangle.

iv) Draw the perpendicular bisector of BC.

v) Draw the angle bisector of angle ACB which intersects the perpendicular bisector of BC at P.

vi) Join PB and draw .

Proof: In  and 

Hence, P is equidistant from B and C.

Again in and 

Hence, P is equidistant from AC and BC.

Question 8

In parallelogram ABCD, side AB is greater than side BC and P is a point in AC such that PB bisects angle B. Prove that P is equidistant from AB and BC.

Solution 8

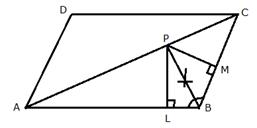
Construction: From P, draw  and 

Proof: In and 

Hence, P is equidistant from AB and BC.

Question 9

In triangle LMN, bisectors of interior angles at L and N intersect each other at point A.

Prove that -

i) point A is equidistant from all the three sides of the triangle.

ii) AM bisects angle LMN.

Solution 9

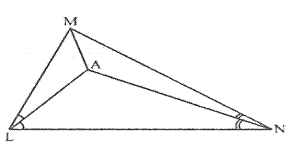
Construction: Join AM

Proof:

A lies on bisector of 

 A is equidistant from MN and LN.

Again, A lies on bisector of 

 A is equidistant from LN and LM.

Hence, A is equidistant from all sides of the triangle LMN.

 A lies on the bisector of 

Question 10

Use ruler and compasses only for this question.

(i)Construct , where AB = 3.5 cm, BC = 6 cm and 

(ii)Construct the locus of points inside the triangle which are equidistant from BA and BC.

(iii)Construct the locus of points inside the triangle which are equidistant from B and C.

(iv)Mark the point P which is equidistant from AB, BC and also equidistant from B and C. Measure and record the length of PB.

Solution 10

Steps of construction:

    (i)Draw line BC = 6 cm and an angle CBX = 60^o. Cut off AB = 3.5. Join AC, triangle ABC is the required triangle.

    (ii)Draw perpendicular bisector of BC and bisector of angle B

    (iii)Bisector of angle B meets bisector of BC at P.

 BP is the required length, where, PB = 3.5 cm

    (iv)P is the point which is equidistant from BA and BC, also equidistant from B and C.

 

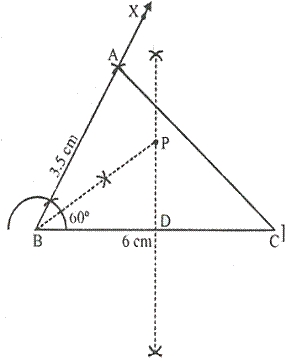
PB=3.6 cm

Question 11

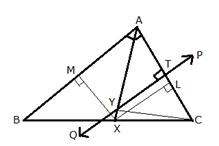
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.

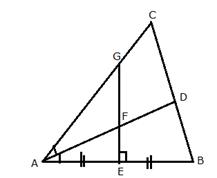
Prove that:

i) F is equidistant from A and B.

ii) F is equidistant from AB and AC.

Solution 11

i)

Construction: Join FB and FC

Proof: In and 

Hence, F is equidistant from A and B.

ii)

Construction: Draw 

Proof: In and 

Hence, F is equidistant from AB and AC.

Question 12

The bisectors of B and C of a quadrilateral ABCD intersect each other at point P. Show that P is equidistant from the opposite sides AB and CD.

Solution 12

Since P lies on the bisector of angle B,

therefore, P is equidistant from AB and BC .... (1)

Similarly, P lies on the bisector of angle C,

therefore, P is equidistant from BC and CD .... (2)

From (1) and (2),

Hence, P is equidistant from AB and CD.

Question 13

Draw a line AB = 6 cm. Draw the locus of all the points which are equidistant from A and B.

Solution 13

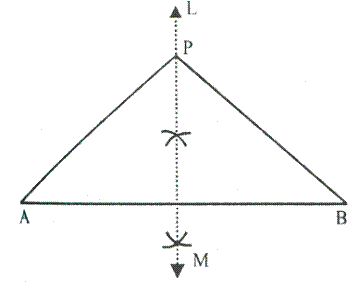
Steps of construction:

(i) Draw a line segment AB of 6 cm.

(ii) Draw perpendicular bisector LM of AB. LM is the required locus.

(iii) Take any point on LM say P.

(iv) Join PA and PB.

Since, P lies on the right bisector of line AB.

Therefore, P is equidistant from A and B.

i.e. PA = PB

Hence, Perpendicular bisector of AB is the locus of all points which are equidistant from A and B.

Question 14

Draw an angle . Draw the locus of all points equidistant from AB and BC.

Solution 14

Steps of Construction:

i) Draw a ray BC.

ii) Construct a ray RA making an angle of with BC. Therefore, .

iii) Draw the angle bisector BP of .

BP is the required locus.

iv) Take any point D on BP.

v) From D, draw and 

Since D lies on the angle bisector BP of 

D is equidistant from AB and BC.

Hence, DE = DF

Similarly, any point on BP is equidistant from AB and BC.

Therefore, BP is the locus of all points which are equidistant from AB and BC.

Question 15

Draw an angle , having AB = 4.6 cm and BC = 5 cm. Find a point P equidistant from AB and BC; and also equidistant from A and B.

Solution 15

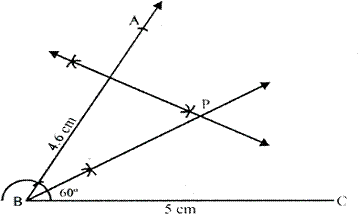
Steps of Construction:

i) Draw a line segment BC = 5 cm

ii) At B, draw a ray BX making an angle of and cut off BA = 4.6 cm.

iii) Draw the angle bisector of .

iv) Draw the perpendicular bisector of AB which intersects the angle bisector at P.

P is the required point which is equidistant from AB and BC, as well as from A and B.

Question 16

In the figure given below, find a point P on CD equidistant from points A and B.

Solution 16

Steps of Construction:

i) AB and CD are the two lines given.

ii) Draw a perpendicular bisector of line AB which intersects CD in P.

P is the required point which is equidistant from A and B.

Since P lies on perpendicular bisector of AB; PA = PB.

Question 17

In the given triangle ABC, find a point P equidistant from AB and AC; and also equidistant from B and C.

Solution 17

Steps of Construction:

i) In the given triangle, draw the angle bisector of .

ii) Draw the perpendicular bisector of BC which intersects the angle bisector at P.

P is the required point which is equidistant from AB and AC as well as from B and C.

Since P lies on angle bisector of ,

It is equidistant from AB and AC.

Again, P lies on perpendicular bisector of BC,

Therefore, it is equidistant from B and C.

Question 18

Construct a triangle ABC, with AB = 7 cm, BC = 8 cm and . Locate by construction the point P such that:

i) P is equidistant from B and C.

ii) P is equidistant from AB and BC.

Measure and record the length of PB.

Solution 18

Steps of Construction:

1) Draw a line segment AB = 7 cm.

2) Draw angle with the help of compass.

3)Cut off BC = 8 cm.

4)Join A and C.

5)The triangle ABC so formed is the required triangle.

i) Draw the perpendicular bisector of BC. The point situated on this line will be equidistant from B and C.

ii) Draw the angle bisector of . Any point situated on this angular bisector is equidistant from lines AB and BC.

The point which fulfills the condition required in (i) and (ii) is the intersection point of bisector of line BC and angular bisector of .

P is the required point which is equidistant from AB and AC as well as from B and C.

On measuring the length of line segment PB, it is equal to 4.5 cm.

Question 19

On a graph paper, draw lines x = 3 and y = -5. Now, on the same graph paper, draw the locus of the point which is equidistant from the given lines.

Solution 19

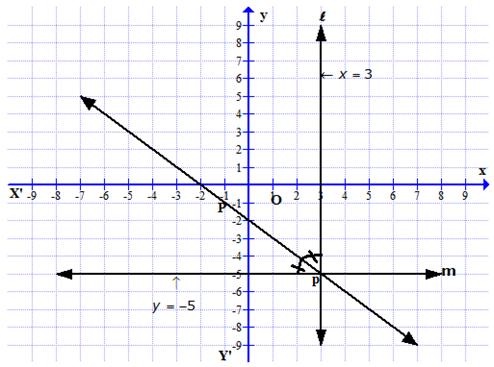
On the graph, draw axis XOX’ and YOY’

Draw a line l, x = 3 which is parallel to y-axis

And draw another line m, y = -5, which is parallel to x-axis

These two lines intersect each other at P.

Now draw the angle bisector p of angle P.

Since p is the angle bisector of P, any point on P is equidistant from l and m.

Therefore, this line p is equidistant from l and m.

Question 20

On a graph paper, draw the line x = 6. Now, on the same graph paper, draw the locus of the point which moves in such a way that its distance from the given line is always equal to 3 units.

Solution 20

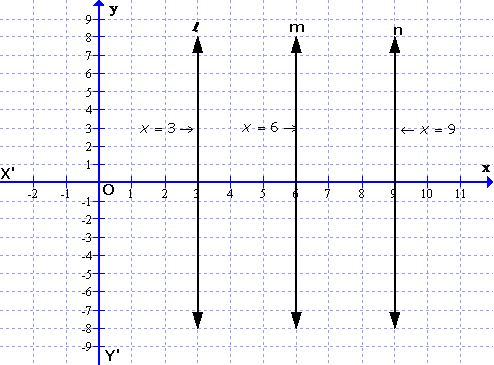
On the graph, draw axis XOX’ and YOY’

Draw a line l, x = 6 which is parallel to y-axis

Take points P and Q which are at a distance of 3 units from the line l.

Draw lines m and n from P and Q parallel to l

With locus = 3, two lines can be drawn x = 3 and x = 9.