If a: b = 5: 3, find: ![]() .
.
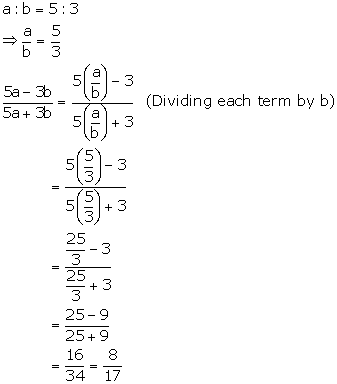
If x: y = 4: 7, find the value of (3x + 2y): (5x + y).

If a: b = 3: 8, find the value of ![]() .
.
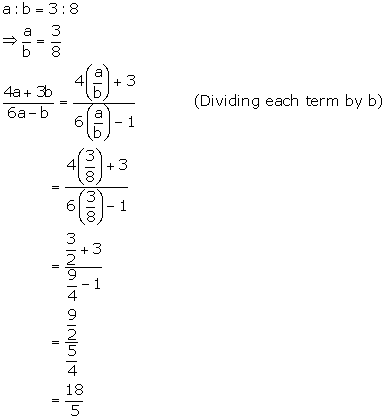
If (a - b): (a + b) = 1: 11, find the ratio (5a + 4b + 15): (5a - 4b + 3).
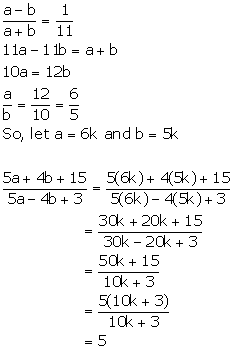
Hence, (5a + 4b + 15): (5a - 4b + 3) = 5: 1
If![]() .
.
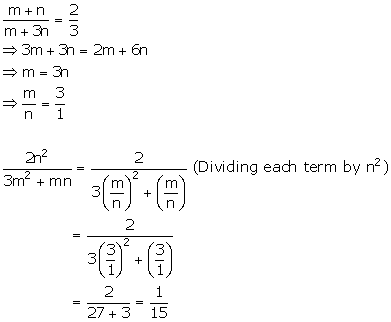
Find![]() , when x2 + 6y2 = 5xy.
, when x2 + 6y2 = 5xy.
x2 + 6y2 = 5xy
Dividing both sides by y2, we get,

Given, 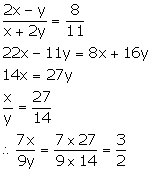
A school has 630 students. The ratio of the number of boys to the number of girls is 3 : 2. This ratio changes to 7 : 5 after the admission of 90 new students. Find the number of newly admitted boys.
What quantity must be subtracted from each term of the ratio 9: 17 to make it equal to 1: 3?
Let x be subtracted from each term of the ratio 9: 17.

Thus, the required number which should be subtracted is 5.
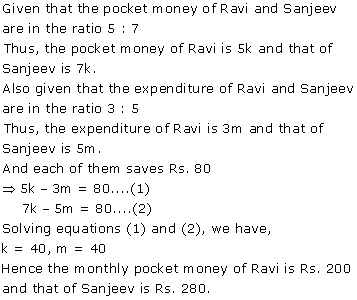
The monthly pocket money of Ravi and Sanjeev are in the ratio 5 : 7. Their expenditures are in the ratio 3 : 5. If each saves Rs. 80 every month, find their monthly pocket money.
The work done by (x - 2) men in (4x + 1) days and the work done by (4x + 1) men in (2x - 3) days are in the ratio 3: 8. Find the value of x.
Assuming that all the men do the same amount of work in one day and one day work of each man = 1 units, we have,
Amount of work done by (x - 2) men in (4x + 1) days
= Amount of work done by (x - 2)(4x + 1) men in one day
= (x - 2)(4x + 1) units of work
Similarly,
Amount of work done by (4x + 1) men in (2x - 3) days
= (4x + 1)(2x - 3) units of work
According to the given information,

The bus fare between two cities is increased in the ratio 7: 9. Find the increase in the fare, if:
(i) the original fare is Rs 245;
(ii) the increased fare is Rs 207.
According to the given information,
Increased (new) bus fare = ![]() original bus fare
original bus fare
(i) We have:
Increased (new) bus fare = ![]() Rs 245 = Rs 315
Rs 245 = Rs 315
![]() Increase in fare = Rs 315 - Rs 245 = Rs 70
Increase in fare = Rs 315 - Rs 245 = Rs 70
(ii) We have:
Rs 207 = ![]() original bus fare
original bus fare
Original bus fare = ![]()
![]() Increase in fare = Rs 207 - Rs 161 = Rs 46
Increase in fare = Rs 207 - Rs 161 = Rs 46
By increasing the cost of entry ticket to a fair in the ratio 10: 13, the number of visitors to the fair has decreased in the ratio 6: 5. In what ratio has the total collection increased or decreased?
Let the cost of the entry ticket initially and at present be 10 x and 13x respectively.
Let the number of visitors initially and at present be 6y and 5y respectively.
Initially, total collection = 10x ![]() 6y = 60 xy
6y = 60 xy
At present, total collection = 13x ![]() 5y = 65 xy
5y = 65 xy
Ratio of total collection = 60 xy: 65 xy = 12: 13
Thus, the total collection has increased in the ratio 12: 13.
In a basket, the ratio between the number of oranges and the number of apples is 7: 13. If 8 oranges and 11 apples are eaten, the ratio between the number of oranges and the number of apples becomes 1: 2. Find the original number of oranges and the original number of apples in the basket.
Let the original number of oranges and apples be 7x and 13x.
According to the given information,
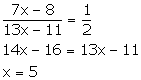
Thus, the original number of oranges and apples are 7 ![]() 5 = 35 and 13
5 = 35 and 13 ![]() 5 = 65 respectively.
5 = 65 respectively.
In a mixture of 126 kg of milk and water, milk and water are in ratio 5 : 2. How much water must be added to the mixture to make this ratio 3 : 2?
(A) If A: B = 3: 4 and B: C = 6: 7, find:
(i) A: B: C (ii) A: C
(B) If A : B = 2 : 5 and A : C = 3 : 4, find
(i) A : B : C
(A)
(i)

(ii)
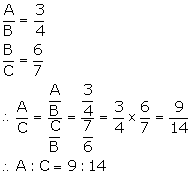
(B)
(i)
If 3A = 4B = 6C; find A: B: C.
3A = 4B = 6C
3A = 4B ![]()
4B = 6C ![]()
Hence, A: B: C = 4: 3: 2
If 2a = 3b and 4b = 5c, find: a : c.
Find the compound ratio of:
(i) 2: 3, 9: 14 and 14: 27
(ii) 2a: 3b, mn: x2 and x: n.
(iii) ![]()
(i) Required compound ratio = 2 ![]() 9
9 ![]() 14: 3
14: 3 ![]() 14
14 ![]() 27
27
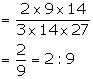
(ii) Required compound ratio = 2a ![]() mn
mn![]() x: 3b
x: 3b ![]() x2
x2![]() n
n
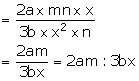
(iii) Required compound ratio = ![]()
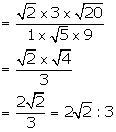
Find duplicate ratio of:
(i) 3: 4 (ii) ![]()
(i) Duplicate ratio of 3: 4 = 32: 42 = 9: 16
(ii) Duplicate ratio of ![]()
Find the triplicate ratio of:
(i) 1: 3 (ii) ![]()
(i) Triplicate ratio of 1: 3 = 13: 33 = 1: 27
(ii) Triplicate ratio of ![]()

Find sub-duplicate ratio of:
(i) 9: 16 (ii) (x - y)4: (x + y)6
(i) Sub-duplicate ratio of 9: 16 = ![]()
(ii) Sub-duplicate ratio of(x - y)4: (x + y)6
= ![]()
Find the sub-triplicate ratio of:
(i) 64: 27 (ii) x3: 125y3
(i) Sub-triplicate ratio of 64: 27 = ![]()
(ii) Sub-triplicate ratio of x3: 125y3 = ![]()
Find the reciprocal ratio of:
(i) 5: 8 (ii) ![]()
(i) Reciprocal ratio of 5: 8 = ![]()
(ii) Reciprocal ratio of 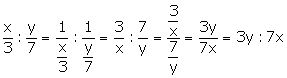
If (x + 3) : (4x + 1) is the duplicate ratio of 3 : 5, find the value of x.
If (x + 3) : (4x + 1) is the duplicate ratio of 3 : 5, find the value of x.
If m: n is the duplicate ratio of m + x: n + x; show that x2 = mn.
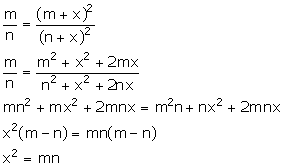
If (3x - 9) : (5x + 4) is the triplicate ratio of 3 : 4, find the value of x.
Find the ratio compounded of the reciprocal ratio of 15: 28, the sub-duplicate ratio of 36: 49 and the triplicate ratio of 5: 4.
Reciprocal ratio of 15: 28 = 28: 15
Sub-duplicate ratio of 36: 49 = ![]()
Triplicate ratio of 5: 4 = 53: 43 = 125: 64
Required compounded ratio
= ![]()
If r2 =pq, show that p : q is the duplicate ratio of (p + r) : (q + r).

No comments:
Post a Comment