Chapter 22 - Heights and Distances Exercise Ex. 22(C)

Question 1

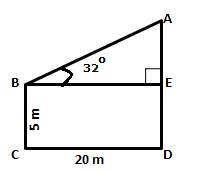
Find AD:

(i)

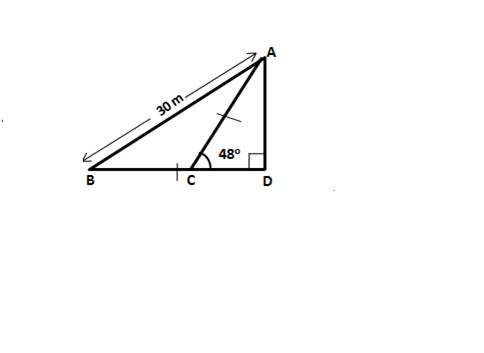
(ii)

Solution 1

Question 2

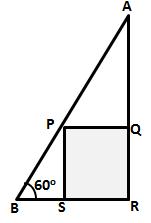
In the following diagram, AB is a floor-board; PQRS is a cubical box with each edge = 1 m and B = 60^o. Calculate the length of the board AB.

Solution 2

Question 3

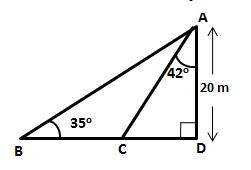
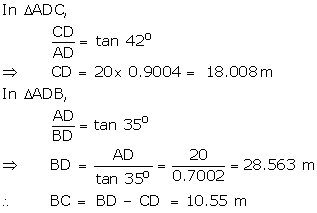
Calculate BC.

Solution 3

Question 4

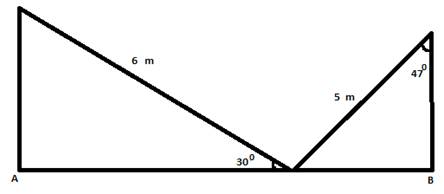
Calculate AB.

Solution 4

 

Question 5

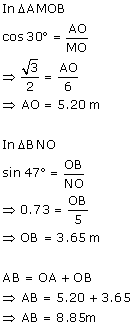
The radius of a circle is given as 15 cm and chord AB subtends an angle of 131^o at the centre C of the circle. Using trigonometry, calculate:

(i) the length of AB;

(ii) the distance of AB from the centre C.

Solution 5

Given, CA = CB = 15 cm, ACB = 131^o

Drop a perpendicular CP from centre C to the chord AB.

Then CP bisects ACB as well as chord AB.

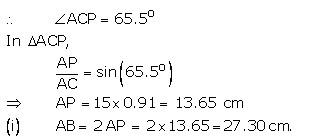
(ii) CP = AC cos (65.5^o)

          =15×0.415 = 6.225 cm.

Question 6

At a point on level ground, the angle of elevation of a vertical tower is found to be such that its tangent is . On walking 192 metres towards the tower, the tangent of the angle is found to be . Find the height of the tower.

Solution 6

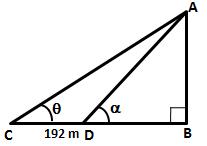
Let AB be the vertical tower and C and D be two points such that CD = 192 m. Let ACB =  and ADB = .

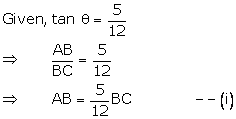
Hence, the height of the tower is 180 m.

Question 7

A vertical tower stands on a horizontal plane and is surmounted by a vertical flagstaff of height h metre. At a point on the plane, the angle of elevation of the bottom of the flagstaff is  and at the top of the flagstaff is . Prove that the height of the tower is .

Solution 7

Let AB be the tower of height x metre, surmounted by a vertical flagstaff AD. Let C be a point on the plane such that  and AD = h.

Question 8

With reference to the given figure, a man stands on the ground at point A, which is on the same horizontal plane as B, the foot of the vertical pole BC. The height of the pole is 10 m. The man's eye s 2 m above the ground. He observes the angle of elevation of C, the top of the pole, as x^o , where tan x^o = . Calculate:

(i) the distance AB in metres;

(ii) angle of elevation of the top of the pole when he is standing 15 metres from the pole. Give your answer to the nearest degree.

Solution 8

Let AD be the height of the man, AD = 2 m.

Question 9

The angles of elevation of the top of a tower from two points on the ground at distances a and b metres from the base of the tower and in the same line are complementary. Prove that the height of the tower is  metre.

Solution 9

Let AB be the tower of height h metres.

Let C and D be two points on the level ground such that BC = b metres, BD = a metres, .

Question 10

From a window A, 10 m above the ground the angle of elevation of the top C of a tower is x^o, where tan x^o =  and the angle of depression of the foot D of the tower is y^o, where tan y^o = . Calculate the height CD of the tower in metres.

Solution 10

Question 11

A vertical tower is 20 m high. A man standing at some distance from the tower knows that the cosine of the angle of elevation of the top of the tower is 0.53. How far is he standing from the foot of the tower?

Solution 11

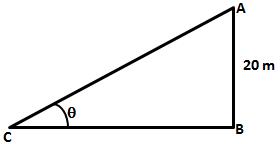
Let AB be the tower of height 20 m.

Let be the angle of elevation of the top of the tower from point C.

Question 12

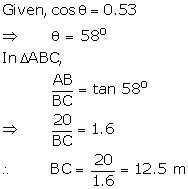
A man standing on the bank of a river observes that the angle of elevation of a tree on the opposite bank is 60^o. When he moves 50 m away from the bank, he finds the angle of elevation to be 30^o. Calculate:

(i) the width of the river;

(ii) the height of the tree.

Solution 12

Let AB be the tree and AC be the width of the river. Let D be a point such that CD = 50 m. Given that 

Question 13

A 20 m high vertical pole and a vertical tower are on the same level ground in such a way that the angle of elevation of the top of the tower, as seen from the foot of the pole is 60^o and the angle of elevation of the top of the pole, as seen from the foot of the tower is 30^o. Find:

(i) the height of the tower ;

(ii) the horizontal distance between the pole and the tower.

Solution 13

Question 14

A vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole, the angle of elevation of the top of the tower is 60^o and the angle of depression of the bottom of the tower is 30^o. Find:

(i) the height of the tower, if the height of the pole is 20 m;

(ii) the height of the pole, if the height of the tower is 75 m.

Solution 14

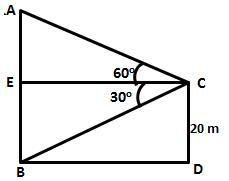
Let AB be the tower and CD be the pole.

Then 

Question 15

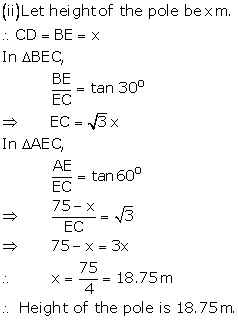
From a point, 36 m above the surface of a lake, the angle of elevation of a bird is observed to be 30^o and the angle of depression of its image in the water of the lake is observed to be 60^o. Find the actual height of the bird above the surface of the lake.

Solution 15

Let A be a point 36 m above the surface of the lake and B be the position of the bird. Let B' be the image of the bird in the water.

Question 16

A man observes the angle of elevation of the top of a building to be 30^o. He walks towards it in a horizontal line through its base. On covering 60 m, the angle of elevation changes to 60^o. Find the height of the building correct to the nearest metre.

Solution 16

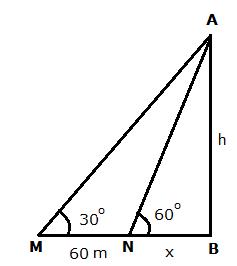
Let AB be a building and M and N are the two positions of the man which makes angles of elevation of top of building as 30^o and 60^o respectively.

MN = 60 m

Let AB = h and NB = x m

Question 17

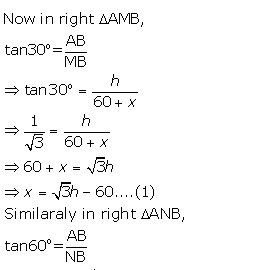
As observed from the top of a 80 m tall lighthouse, the angles of depression of two ships, on the same side of a light house in a horizontal line with its base, are 30° and 40° respectively. Find the distance between the two ships. Give your answer corrected to the nearest metre.

Solution 17

Let AB represent the lighthouse.

Let the two ships be at points D and C having angle of depression 30° and 40° respectively.

Let x be the distance between the two ships.

The distance between the two ships is 43 m.

Question 18

In the given figure, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find :

(i) the horizontal distance between AB and CD.

(ii) the height of the lamp post.

 

 

Solution 18

 

 

Question 19

An aeroplane, at an altitude of 250 m, observes the angles of depression of two boats on the opposite banks of a river to be 45° and 60° respectively. Find the width of the river. Write the answer correct to the nearest whole number.

Solution 19

Let A be the position of the airplane and let BC be the river. Let D be the point in BC just below the airplane.

B and C be two boats on the opposite banks of the river with angles of depression 60° and 45° from A.

Question 20

The horizontal distance between two towers is 120 m. The angle of elevation of the top and angle of depression of the bottom of the first tower as observed from the top of the second tower is 30° and 24° respectively. Find the height of the two towers. Give your answers. Give your answer correct to 3 significant figures.

 

 

Solution 20

 

Question 21

The angles of depression of two ships A and B as observed from the top of a light house 60m high, are 60° and 45° respectively. If the two ships are on the opposite sides of the light house, find the distance between the two ships. Give your answer correct to the nearest whole number.

Solution 21

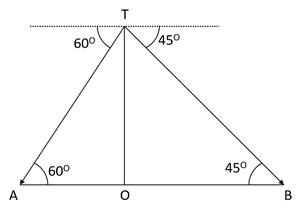
In the above figure

OT=tower = 60m

A and B are the respective positions of ship