From a solid right circular cylinder with height 10 cm and radius of the base 6 cm, a right circular cone of the same height and same base are removed. Find the volume of the remaining solid.
Height of the cylinder (h) = 10 cm
and radius of the base (r) = 6 cm
Volume of the cylinder = 
Height of the cone = 10 cm
Radius of the base of cone = 6 cm
Volume of the cone = 
Volume of the remaining part
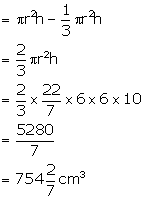
From a solid cylinder whose height is 16 cm and radius is 12 cm, a conical cavity of height 8 cm and of base radius 6 cm is hollowed out. Find the volume and total surface area of the remaining solid.
Radius of solid cylinder (R) = 12 cm
and Height (H) = 16 cm
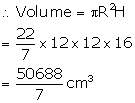
Radius of cone (r) = 6 cm, and height (h) = 8 cm.
(i) Volume of remaining solid
(ii) Slant height of cone 
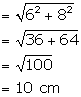
Therefore, total surface area of remaining solid = curved surface area of cylinder + curved surface area of cone + base area of cylinder + area of circular ring on upper side of cylinder
A circus tent is cylindrical to a height of 4 m and conical above it. If its diameter is 105 m and its slant height is 80 m, calculate the total area of canvas required. Also, find the total cost of canvas used at Rs 15 per meter if the width is 1.5 m
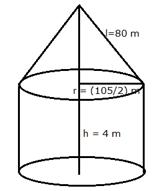
Radius of the cylindrical part of the tent (r) = 
Slant height (
) = 80 m
Therefore, total curved surface area of the tent = 
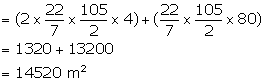
Width of canvas used = 1.5 m
Length of canvas = 
Total cost of canvas at the rate of Rs 15 per meter
A circus tent is cylindrical to a height of 8 m surmounted by a conical part. If total height of the tent is 13 m and the diameter of its base is 24 m; calculate:
(i) total surface area of the tent
(ii) area of canvas, required to make this tent allowing 10% of the canvas used for folds and stitching.
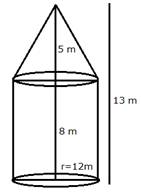
Height of the cylindrical part = H = 8 m
Height of the conical part = h = (13-8)m = 5 m
Diameter = 24 m 
radius = r = 12 m
Slant height of the cone = l
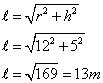
Slant height of cone = 13 m
(i) Total surface area of the tent = 
(ii)Area of canvas used in stitching = total area
A cylindrical boiler, 2 m high, is 3.5 m in diameter. It has a hemispherical lid. Find the volume of its interior, including the part covered by the lid.
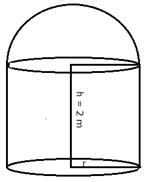
Diameter of cylindrical boiler = 3.5 m
Height (h) = 2 m
Radius of hemisphere (R) = 
Total volume of the boiler = 
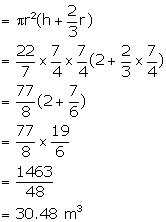
A vessel is a hollow cylinder fitted with a hemispherical bottom of the same base. The depth of the cylindrical part is 
and the diameter of hemisphere is 3.5 m. Calculate the capacity and the internal surface area of the vessel.
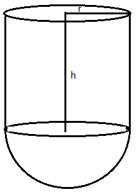
Diameter of the base = 3.5 m
Therefore, radius = 
Height of cylindrical part = 
(i) Capacity (volume) of the vessel = 
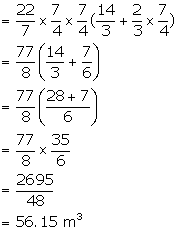
(ii)Internal curved surface area = 
A wooden toy is in the shape of a cone mounted on a cylinder as shown alongside.
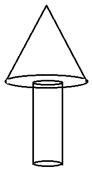
If the height of the cone is 24 cm, the total height of the toy is 60 cm and the radius of the base of the cone = twice the radius of the base of the cylinder = 10 cm; find the total surface area of the toy. [Take 
]
Height of the cone = 24 cm
Height of the cylinder = 36 cm
Radius of the cone = twice the radius of the cylinder = 10 cm
Radius of the cylinder = 5 cm
Slant height of the cone = 
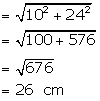
Now, the surface area of the toy = curved area of the conical point + curved area of the cylinder
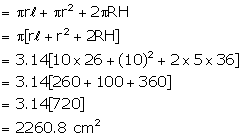
A cylindrical container with diameter of base 42 cm contains sufficient water to submerge a rectangular solid of iron with dimensions 22 cm 
14 cm 10.5 cm. Find the rise in level of the water when the solid is submerged.
Diameter of cylindrical container = 42 cm
Therefore, radius (r) = 21 cm
Dimensions of rectangular solid = 22cm 14cm 10.5cm
Volume of solid = 
Let height of water = h
Therefore, volume of water in the container = 
From (i) and (ii)
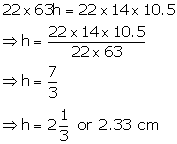
Spherical marbles of diameter 1.4 cm are dropped into beaker containing some water and are fully submerged. The diameter of the beaker is 7 cm. Find how many marbles have been dropped in it if the water rises by 5.6 cm.
Diameter of spherical marble = 1.4 cm
Therefore, radius = 0.7 cm
Volume of one ball 
Diameter of beaker = 7 cm
Therefore, radius = 
Height of water = 5.6 cm
Volume of water = 
No. of balls dropped
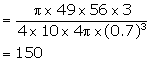
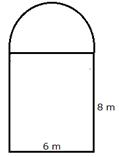
The cross-section of a railway tunnel is a rectangle 6 m broad and 8 m high surmounted by a semi-circle as shown in the figure.
The tunnel is 35 m long. Find the cost of plastering the internal surface of the tunnel (excluding the floor) at the rate of Rs 2.25 per m2.
Breadth of the tunnel = 6 m
Height of the tunnel = 8 m
Length of the tunnel = 35 m
Radius of the semi-circle = 3 m
Circumference of the semi-circle = 
Internal surface area of the tunnel
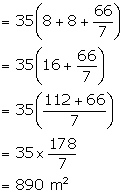
Rate of plastering the tunnel = Rs 2.25 per m2
Therefore, total expenditure 
The horizontal cross-section of a water tank is in the shape of a rectangle with semicircle at one end, as shown in the following figure.
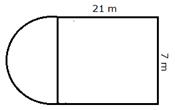
The water is 2.4 metres deep in the tank. Calculate the volume of water in the tank in gallons. (Given: 1 gallon = 4.5 litres)
Length = 21 m
Depth of water = 2.4 m
Breadth = 7 m
Therefore, radius of semicircle = 
Area of cross-section = area of rectangle + Area of semicircle
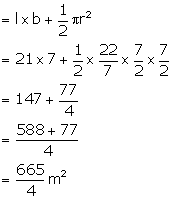
Therefore, Volume of water filled in gallons
The given figure shows the cross-section of a water channel consisting of a rectangle and a semicircle. Assuming that the channel is always full, find the volume of water discharged through it in one minute if water is flowing at the rate of 20 cm per second. Give your answer in cubic meters correct to one place of decimal.
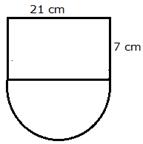
Length = 21 cm, Breadth = 7 cm
Radius of semicircle = 
Area of cross section of the water channel = 
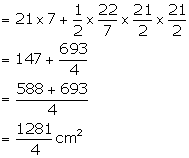
Flow of water in one minute at the rate of 20 cm per second
Length of the water column = 20 
60 = 1200 cm
Therefore, volume of water =
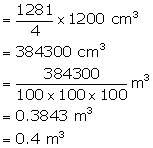
An open cylindrical vessel of internal diameter 7 cm and height 8 cm stands on a horizontal table. Inside this is placed a solid metallic right circular cone, the diameter of whose base is 
cm and height 8 cm. Find the volume of water required to fill the vessel. If this cone is replaced by another cone, whose height is 
cm and radius of whose base is 2 cm, find the drop in the water level.
Diameter of the base of the cylinder = 7 cm
Therefore, radius of the cylinder = 
Volume of the cylinder 
Diameter of the base of the cone =
Therefore, radius of the cone = 
Volume of the cone 
On placing the cone into the cylindrical vessel, the volume of the remaining portion where the water is to be filled
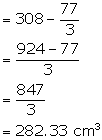
Height of new cone = 
Radius = 2 cm
Therefore, volume of new cone
Volume of water which comes down = 
Let h be the height of water which is dropped down.
Radius =
From (i) and (ii)
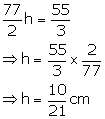
Drop in water level = 
A cylindrical can, whose base is horizontal and of radius 3.5 cm, contains sufficient water so that when a sphere is placed in the can, the water just covers the sphere. Given that the sphere just fits into the can, calculate:
(i) the total surface area of the can in contact with water when the sphere is in it;
(ii) the depth of water in the can before the sphere was put into the can.
Radius of the base of the cylindrical can = 3.5 cm
(i) When the sphere is in can, then total surface area of the can =
Base area + curved surface area
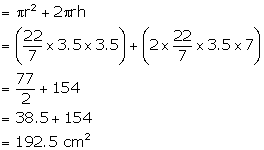
(ii) Let depth of water = x cm
When sphere is not in the can, then volume of the can =
volume of water + volume of sphere
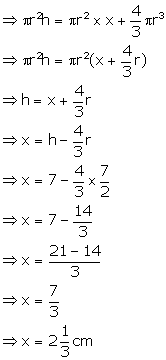
A hollow cylinder has solid hemisphere inward at one end and on the other end it is closed with a flat circular plate. The height of water is 10 cm when flat circular surface is downward. Find the level of water, when it is inverted upside down, common diameter is 7 cm and height of the cylinder is 20 cm.
Let the height of the water level be 'h', after the solid is turned upside down.
Volume of water in the cylinder
Volume of the hemisphere
Volume of water in the cylinder
= Volume of water level - Volume of the hemisphere
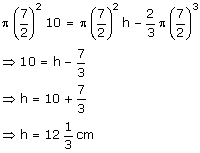
No comments:
Post a Comment